Skip to main content

The quantum nonlocality and Monte Carlo methodology are the ultimate tools in the quantum system's theoretical manipulation.


The complexity is one problem of quantum systems. The quantum system is the entirety where different types of power fields, along with the particles interact with each other. Even if we see two different electrons or pair of two similar-looking elementary particles those particles might not be identical. 

Their energy levels might be different and that makes a difference between them. So even if we see a simple-looking structure, it can hide a very complex quantum structure. 

Manipulation of the system requires knowledge of the entire system. Quantum technology allows the aiming of energy impulses into individual particles, that form the system. The problem in quantum technology is quantum nonlocality.

We can say that quantum nonlocality means that. We cannot interact with one individual actor in the quantum system. When we send energy impulses to the quantum system. That energy will escalate into the entire system.

 So if we will send energy into the quantum system, that thing always interacts with the entire system. Another problem is: how to adjust the energy that the quantum system impacts into the targeted systems.

We know how to impact energy to the system. But we don't know how to remove energy with the very high accuracy needed in quantum-scale technology. 


In Wikipedia, the explanation of quantum nonlocality goes like this: 

"In theoretical physics, quantum nonlocality refers to the phenomenon by which the measurement statistics of a multipartite quantum system do not admit an interpretation in terms of a local realistic theory. " (Wikipedia/Quantum nonlocality)

"Quantum nonlocality has been experimentally verified under different physical assumptions. Any physical theory that aims at superseding or replacing quantum theory should account for such experiments and therefore cannot fulfill local realism; quantum nonlocality is a property of the universe that is independent of our description of nature." (Wikipedia/Quantum nonlocality)



"Entangled quantum objects can be used to network separated systems. The researchers demonstrate what is needed for nonlocal correlations, a requirement for a useful quantum network. Credit: The Grainger College of Engineering at the University of Illinois Urbana-Champaign/Wesley Moore" (ScitechDaily.com/Decoding Quantum Nonlocality: A New Criterion for Quantum Networks)


Successful quantum manipulation requires high-standard simulations. 




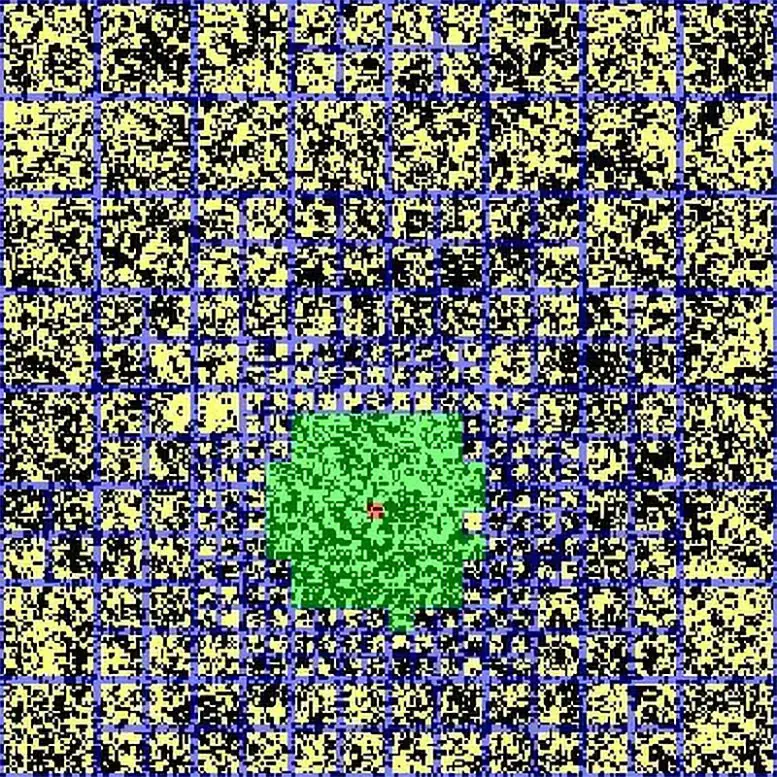
"Visualization of the process to calculate the new state of the spin (shown in red) of a ferromagnetic system with long-range interactions. The near-field region (green) is treated as for short-range interactions, while in the far-field region (yellow) hierarchical data structures (size of the blue boxes) are used that are adapted to the instantaneous system state. Credit: Institute of Theoretical Physics and Leipzig University" (ScitechDaily.com/From Centuries to Days: Breakthrough in Monte Carlo Computer Simulations)




"Monte Carlo method applied to approximating the value of π." (Wikipedia/Monte Carlo method)


The animation shows the cycle of the cases. If we think about theoretical principles. The Monte Carlo simulation is very easy to make. It's the successful cases that divide all cases. (Successful cases/all cases). But in things like neutron diffusion calculations, there are so many cases, that it makes calculations very complicated. And forward this text I will explain, why it's so hard to calculate possibility. That asteroid impacts on Earth. 


"Leipzig University researchers have developed a highly efficient method and algorithm for studying long-range interaction systems. The algorithm dramatically reduces computational time, offering profound insights into nonequilibrium processes. This breakthrough has vast implications for both theoretical research and practical applications." (ScitechDaily.com/From Centuries to Days: Breakthrough in Monte Carlo Computer Simulations)




The breakthrough in the Monte Carlo method


Successful cases/ possible cases are the thing that is used to calculate how many randomly happening cases are involved in entirety. In mathematics, the Monte Carlo method or Monte Carlo simulation is how many successful cases involving in the full count of possible cases. 

One of the simplest examples is when a person throws a ball into holes when there is only one ball for each hole. And then the person calculates how many of those cases are successful. In some of those examples person's eyes are tied. But there are many other versions of how to confess to people about randomness. 

So the calculation goes like this: The number of successful cases will be divided by the number of possible cases. The term "Monte Carlo" method is known to some people from nuclear energy and nuclear fission. In those cases, that term means how many percents of the neutrons that fissile atoms send impact with other atoms. 

The calculated hits of neutrons divide by the number of possible atoms. The Monte Carlo method was invented by Enrico Fermi when he calculated neutron diffusion. The idea is similar to roulette. Each number in the roulette plate has a certain area. And there is a possibility, that we can calculate that the ball hits a certain number. 

Today the University of Leipzig's researchers are making algorithms that can improve the accuracy of the Monte Carlo simulation. That thing helps them to understand interactions between systems better. As you saw before. The principle of the Monte Carlo method is simple. 


Why it's so difficult to calculate asteroids' trajectories? 


If we want to calculate the possibility that some meteorite hits a person we must just calculate the land area that the person uses and divide it by using the Earth's surface area. The problem is how to calculate the possibility that a meteorite hits Earth. 

At that moment, we must realize that also other forces than gravitation. Interact with that meteorite. The gravitational fields can affect the object. But also things like electromagnetic fields interact with iron and magnetic minerals.

The solar wind can also affect the trajectories of meteorites as well as ice on their shells. When the sun's heat affects that ice, the radiation from the sun vaporizes that ice. Forms a reaction effect that can push meteorite out from its track. 

So even if we can calculate values of all quantum fields at the precise point in our solar system, we cannot calculate individual asteroid trajectories. Things like flares are making those quantum fields unstable. To make that thing we must know the entire system. The knowledge of the quantum field's power is not enough. We must know all interactions that can affect the particle. 


https://scitechdaily.com/from-centuries-to-days-breakthrough-in-monte-carlo-computer-simulations/


https://scitechdaily.com/decoding-quantum-nonlocality-a-new-criterion-for-quantum-networks/


https://en.wikipedia.org/wiki/Diffusion

https://en.wikipedia.org/wiki/Monte_Carlo_method

https://en.wikipedia.org/wiki/Quantum_nonlocality


Comments

Post a Comment

Popular posts from this blog

Black holes cause a virtual redshift because gravitation stretches the wavelength near them.

At the beginning of this text is a film about the redshift of black holes. Gravitation stretches light, and that means gravitation fields are pulling waves longer. That thing is called the gravitational redshift. As you can see from the film, the black hole stretches radiation and distorts the redshift. Gravitational redshift, or virtual redshift, means that a black hole might seem to be at a longer distance than it is. The film shows the redshift of the star that orbits a supermassive black hole. But all other black holes interact the same way.  The event horizon is always constant. At that point, the black hole's escaping velocity is the same as the speed of light. So every black hole interacts basically in the same way. And it's possible to apply that model to all black holes irrespective of their size.  Is gravitation the thing that forms dark energy? That thing seems somehow strange. But when photons and other particles are traveling through the ball that forms the visible
Post a Comment
Read more

The shape of the brain means more than neuro connectivity.

Well, we might say that the brain is in its entirety. Another thing is that all things in the brain have some kind of purpose. The shape of the brain and, especially, the folding of the brain shell are extremely important things. Those folds are expanding the brain's surface areas. And the brain shell has a primary role in the thinking process. The surface area of the brain determines how large the cerebral cortex is. And in a large cerebral cortex, there are a large number of neurons. But as I just wrote, the brain is in its entirety. "Researchers have discovered that the shape of a person’s brain significantly impacts thought, feeling, and behavior, overturning the prevailing emphasis on complex neuronal connectivity. Utilizing MRI scans and the principle of eigenmodes, they found that brain function is closely linked to its geometric properties, much like how the shape of a musical instrument determines its sound, offering new avenues for exploring brain function and diseas
Post a Comment
Read more

New nanomaterial is 4 times harder than steel. And, at the same time 5 times lighter than steel.

 New nanomaterial is 4 times harder than steel. And, at the same time 5 times lighter than steel.  The new material is the hollow glass fiber with DNA molecules inside that structure. Or as you see from the image. The glass fibers are on both sides of the DNA.  DNA molecule is the thing, that involves the genetic code of the cells. Genetically engineered cells can make DNA, and those molecules can used as the nanomaterial's structures. DNA manipulation makes it possible to create new types of extremely strong materials. And those materials are stronger than steel and lighter than it. DNA molecules can act as nano-size springs.  And in some visions, genetically engineered cyborg cells like cyborg macrophages can make extremely long DNA molecules. And then they can just use those molecules as spears that can pierce wanted cells. Or those cyborg cells can also dumb targeted cells full of the DNA that terminates them immediately.  The DNA and nanotube combinations can also act as DNA-b
Post a Comment
Read more